A secondary beam of radioactive ¹⁷F was produced at the ATLAS accelerator and delivered to an experimental target station with an intensity of at least 2·10⁵ particles per second for use in the research program. The beam was produced through the p(¹⁷O,¹⁷F)n inverse reaction by bombarding a hydrogen gas target with 250 particle nA of 83 MeV ¹⁷O from the ATLAS superconducting linac. The gas target was maintained at a pressure of 300 Torr and a temperature of 257K. Beam quality was dominated by multiple scattering in the gas cell windows and by the reaction kinematics and beamline acceptance for energy spread.

Radioactive beams have many applications in modern nuclear physics and astrophysics. This potential has caused several accelerator laboratories around the world to develop techniques to produce radioactive beams with useful intensities and controllable beam properties. The effort to develop a ¹⁷F beam at the superconducting linac ATLAS [1] is primarily aimed at measurements of interest in astrophysics, but is also a demonstration of a technique to produce on-line, short half-life radioactive beams, and deliver these to a secondary target.

The physics goal of the experiment was to measure the cross-section for ¹⁷F(p,a)¹⁴O in the 3 to 4 MeV region in the center of mass system. For this measurement, the inverse reaction p(¹⁷F,¹⁴O)a is appealing, because a target of ¹⁷F (T_½ = 65s) is not possible. For such a short half-life, the batch transfer process, as has been used for radioactive beams ¹⁸F (T_½ = 109m) [2,3], is also impractical. Therefore, a primary proton target was used to transform a fraction of an ¹⁷O beam to a ¹⁷F beam. A bending magnet selected the fluorine particles in charge state 9+, thereby filtering out most of the ¹⁷O isobar nuclei. Finally, the ¹⁷F beam was delivered over a distance of 12 m to a secondary CH₂ target. The desired ¹⁷F beam energy for the 3.63 MeV resonance in inverse kinematics is 62 to 63 MeV, producing approximately 45 MeV ¹⁴O and 15 MeV ⁴He. Since p(¹⁷F,¹⁴O)a is forward peaked in the laboratory system, the ATLAS spectrograph was used as a powerful tool to separate the ¹⁴O nuclei from the background of scattered particles.

Severe challenges had to be overcome in order to carry out a successful measurement with this technique:

• Build a sufficiently robust proton production target
• Transport the ¹⁷F beam of huge energy spread and divergence with maximum efficiency to the target
• Understand and handle beam impurities
• Identify clearly ¹⁴O in the spectrograph in the presence of different backgrounds.

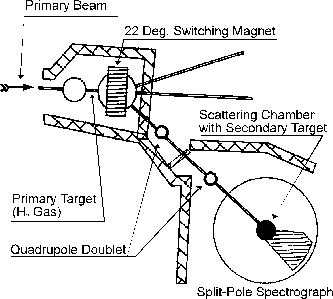
The physical layout showing the relationship of the ¹⁷F production target, the connecting beamline to the secondary target and the ATLAS spectrograph is shown in Fig. 1.

Design of the ¹⁷F Production Target

The ¹⁷F production target must be capable of sustaining an ¹⁷O beam current of as much as one particle mA and have an effective thickness of at least 250 mg/cm², in order to obtain more than 10⁵ particles per second on the secondary target given the ¹⁷F production cross-section [4] in the order of 10 to 100 mb and a transport efficiency of about one percent.

Experience indicates that foil (CH₂) targets cannot take the necessary high beam current, even in rapid rotation. Therefore, a gas target with an effective length of 7.5cm and thin HAVAR [5,6] windows was chosen [see Fig. 2.]. To keep the temperature of both the windows and the hydrogen gas low, the chamber has double walls to accomodate a constantly flowing cooling liquid in the outer cylinder. Four support pipes supply cooling fluid and H₂ gas.

The selection of window material and thickness was made as a compromise between the maximum sustainable gas pressure and the deleterious effect of thick windows on angular and energy straggling. The effect on the energy spread is negligible, since usually the reaction kinematics dominates this even near the reaction threshold. On the other hand, the contribution of the small-angle scattering to the beam divergence can dominate the effective beam emittance. The required energy on the secondary target led to reaction energies between 73 to 77 MeV (threshold at 63.8 MeV) resulting in an maximum divergence of ±1.5° from kinematics (average of about ±1°). This relatively small cone is due to the negative Q-value of p(¹⁷O, ¹⁷F)n. A reaction with a positive Q-value would result in a much larger angular spread.

For two 1.9 mg/cm² HAVAR windows, the small angle scattering is also of the order of ±1°, which made this type of window a good choice. Glued in a mounting ring with an inner diameter of 1.27 cm, they withstood a 250 pnA beam of 83 MeV ¹⁷O at H₂ pressures up to of 460 Torr. A higher pressure was not tested.

The divergence and energy width of the ¹⁷F is estimated to be ±1.6° and ±4 MeV from the combined contribution of all effects. Calculations of the beam optics and examination of the windows after the run suggest a beam spot radius of 2 mm on the entrance windows. This makes a spot with a 3 mm radius on the exit windows. From this, we estimate the unnormalized emittance of the secondary ¹⁷F to be 84 p·mm·mrad.

Transport of the ¹⁷F beam to the secondary target

The program TRANSPORT [7] was used to calculate the beam optics of the ¹⁷F and predict a transport efficiency. For such a large emittance beam, a transmission of 3.5% is predicted, assuming a uniform density profile. Using a peaked density profile increases this number by a factor of 2. It is also estimated that 35% [8] of the ¹⁷F beam is in the 9⁺ charge state, resulting in another attenuation factor of 3. The calculated beam envelope is shown in Fig. 3. and compared to mechanical apertures in the system.

Both energetic and angular distributions of the ¹⁷F beam are strongly affected by the reaction kinematics. Therefore, a correlation between energy and angle of a particle is to be expected. These correlations are not included in the TRANSPORT studies and place significant uncertainties on the predicted transmission. However, this simple model leads to an expected overall efficiency of 1 to 2%.

At a primary beam of 250 pnA ¹⁷O, one expects a ¹⁷F production rate of 2·10⁷ per second. At the spectrograph, a ¹⁷F current of 1·10⁵ per second was observed. The upper limit of the vertical spot size on the secondary target is calculated to be 1cm. The y-magnification of the spectrograph is three, and so the detector [9] in the spectrograph focal plane is unable to intercept all beam particles. We estimate a detection efficiency of only 50% due to this effect, resulting in a transport efficiency of 1%, in reasonable agreement with the estimate in the last paragraph.

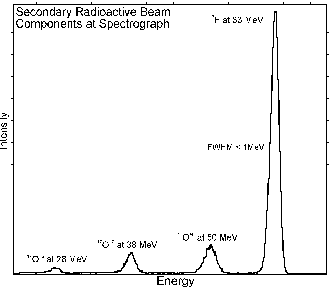
The ratio of ¹⁷F particles to other nuclei detected in the focal plane of the spectrograph in the experiment was better than 3:1, in a measurement taken at 0° and therefore with a much weaker primary ¹⁷O beam of only 175ppA on the gas target [See Fig. 4].

The goal of the experiment was to detect ¹⁴O nuclei from ¹⁷F(p,a)¹⁴O in inverse kinematics. To provide easy discrimination between ¹⁴O nuclei and background, the a particle was detected in coincidence in a silicon detector. While the spectrograph covered 2° to 10° on the left side of the beam, the alpha detector measured a-particles on the right side between 6° and 20°. In the coincidence spectrum, the ¹⁴O particles are the most prominent group [see Fig. 5]. The ¹⁷F and ¹⁷O particles scattered in the spectrograph are only in random coincidence with uncorrelated particles in the silicon detector.

By slightly changing the field of the 22° switching magnet, a certain energy control of the secondary beam was available without retuning the accelerator. Due to the large energy spread in the ¹⁷F particles, the current of the secondary beam did not change more than 50% when swept over a 2 MeV energy region.

This first experiment with a radioactive ¹⁷F-beam shows that a gas target can be used to produce a radioactive beam from a stable beam in flight. However, it is difficult to transport the secondary beam, and the accessible energy range is restricted by the cross-section and the reaction kinematics. Nevertheless, the technique works well, producing on the order of a few 10⁵ particles per second. With this radioactive beam, it is already possible to measure an astrophysically significant nuclear reaction cross section.

To gain a larger range of accessible energies, to uncouple the production reaction energy from the energy of the secondary beam, and to reduce the energy spread of the secondary beam, the production target has to be moved in front of active elements of the accelerator system. A strong focusing element, directly after the gas target also improves the overall transport efficiency. However, the acceptance of the rest of the beam transport system limits this effect.

Testing different production target locations and the refinement of the gas target itself are the next steps in the development of this in-beam production of radioactive beams at ATLAS..

This work was supported by the US D.O.E., Nuclear Science Division, under contract No. W-31-109-ENG-38.

Boris Harss is a student of Prof. H.J. Körner at the Institute for Nuclear Physics and Nuclear Solid State Physics E12, Technische Universität München, working on this project in partial fulfillment for a diploma thesis.

[1] L.M. Bollinger, et al., Nucl. Inst. and Meth. A328, (1993) 211.

[2] R.Coszach, et al., Phys. Lett. B353, (1995) 184.

[3] A.D.Roberts, et al., Nucl. Inst. and Meth., B103 (1995) 523.

[4] J.K.Blair, Phys. Rev. C8, (1), (1973) 120.

[5] L.S. Skaggs, et al., Proc. of the World Conf. of the Internatzional Nucl. Target Development Society, Bosten, MA, USA (1979) 249.

[6] J.D. Carlson, Nucl. Inst. and Meth. 113 (1973) 541.

[7] K.L. Brown, Advances Particle Phys. 1, (1967) 71.

[8] K.Shima, et al., Atomic Data and Nuc. Data Tables, 34 (3) (1986) 358.

[9] K.E.Rehm, F.L.H. Wolfs, Nucl. Inst. and Meth., A273 (1988) 262.