The dielectric response of a charged particle beam
to a periodic focusing field enhances the effective focusing strength,
reducing the matched beam radius and affecting the motion of halo
particles. The change in the effective focusing strength is found
for a uniform-density beam with a diffuse halo in a quadrupole
channel, giving increases of 2% to 8% for some typical examples.
These changes are important for both the production and behavior
of halos in intense, high energy beams, in which fractional current
losses as small as 10-8
/m can result in radioactivation.
The effective focusing strength of a periodic channel is an important factor for accelerator applications requiring high beam intensities, such as heavy ion inertial fusion, radioactive waste transmutation, spallation neutron sources, tritium production and muon production. Limiting currents have been found in the past using the smooth approximation [1] to find the effective focusing strength of a periodic channel which, along with the aperture, determines the current that can be transported through a given channel [2]. Accurate knowledge of the effective focusing strength is also important for matching. Transverse mismatch has been shown to be an important cause of halo production and the resulting particle losses [3-5]. Fractional current losses as small as 10-8 /m can result in radioactivation, inhibiting routine maintenance [6]; this can also be the limiting factor in the transport of intense, high-energy beams [7].
The dielectric response of a plasma to the periodic field of a Paul trap was recently shown to enhance the effective focusing strength of the trap [8]. The dielectric response results from the correlation between the oscillations in the space charge field and the peiodic focusing field [9]. The dielectric response is shown here to increase the effective focusing strength of the channel, by an amount that depends on the shape of the beam, the type of focusing, and the ratio of the plasma frequency of the beam, wp, to the frequency of the focusing, w. The dielectric response and the fractional change in the effective focusing strength are found for a uniform-density continuous beam with a diffuse halo and for a uniform-density ellipsoidal (bunched) beam, both in a quadrupole channel. The increase in the effective focusing strength results in a higher transverse phase advance per period, a higher average beam density and a lower average beam radius. Since accurate matching is important for beam applications requiring low losses, the effect of the dielectric response on the matched beam parameters can be important for the applications listed above.
A beam in a periodic focusing channel experiences a fluctuating electric field Ef (r,s0), which consists of the fluctuating component of the focusing field, Ecf (r, s0), and small fluctuations in the space charge field, Esf (r, s0). The position relative to the center of the beam is r, and the focusing is periodic in s, the longitudinal distance along the channel. Although particles with different longitudinal positions within the beam are at different phases in the periodic field, it is assumed that the effects of this are negligible so that the fluctuating fields can be written as periodic functions of the longitudinal position of the beam center along the channel, s0. The focusing field and the space charge field are each divided into two parts so that the fluctuating components have an average value of zero and the steady-state components vary slowly or not at all with s0.
The frequency of the focusing is w=2pvB/S, where S is the period of the focusing along the longitudinal direction and vB is the beam velocity. In general there are three periods (Sx, Sy and Sz) and three frequencies (wx, wy and wz), one for each of three directions in Cartesian coordinates (x and y are transverse and z is parallel to the beam axis; for most practical applications Sx = Sy). The focusing field can be the result of electrostatic or magnetic quadrupole lenses, induction-acceleration gaps, and magnetic solenoids (if the beam is considered in the Larmor frame). It can also be the result of focusing by electromagnetic fields which are periodic in time and space, as in the case of radio-frequency quadrupole (RFQ) focusing. The focusing field is written as an electric field with the approximation that particle motion in the beam frame is nonrelativistic, so that magnetic focusing can be represented by equivalent electrostatic fields. The force resulting from the magnetic field of the beam is included in the self electric field (the space charge field) with the same approximation. Unless otherwise stated, all quantities are considered in the lab frame. With RFQ focusing and induction-acceleration gaps, it is assumed that acceleration along the longitudinal direction is slow enough that it can be treated as adiabatic, and that the beam is in phase with the time-varying field so that the focusing field can be treated as periodic only in longitudinal distance along the channel.
The effective focusing field can be found from the average field of a particle due to its motion in the periodic field [10]. The motion of a particle in the periodic field is first found with the fluctuating field as a function of position fixed at Ef (r,s0) = Ef (r0,s0), where r0 is the position of the particle averaged over a period. The resulting particle position is r0 + dr; the first-order variation in the position of the particle resulting from the fluctuating field is dr. The effective field that results from the fluctuating field is then found to first order from
![]() (1)
(1)
where DEL0 is the gradient with respect to r0 and the brackets represent averages over a focusing period. The effective field of Equation (1) has previously been derived for a Paul trap without space charge [10] and for a periodic focusing channel without space charge fluctuations [11]. Solving for dr from the fluctuating field and substituting into Equation (1) gives an effective field of
 (2)
(2)
where q and m are respectively the particle charge and mass, and g = (1-vB2/c2)-1/2 is the relativistic factor. In a quadrupole channel the steady-state component of the transverse focusing field is zero, so the field of Equation (2) is the total effective focusing field. For transverse focusing by solenoids or longitudinal focusing by induction-acceleration gaps, the steady-state component of the focusing field is typically much larger than the effective field of Equation (2), so that the dielectric response, which affects only the fluctuating component of the field, has much less effect than in a quadrupole channel with the same frequency and focusing strength.
The dielectric response occurs through the effect of space charge fluctuations on Ef (r0,s0). This will be found first for the core and halo of a uniform-density continuous beam with a diffuse halo in a quadrupole channel with average axial symmetry. The dielectric response will then be considered for the core of a uniform-density ellipsoidal (bunched) beam in a quadrupole channel with average axial symmetry.
The electric field in a transverse direction (x) of a continuous, uniform elliptic beam with current I and velocity vB is Esx = Ix/(pe0g2vBxm(xm+ym)), where xm and ym are respectively the beam envelopes in the x and y directions, and e0 is the permittivity of free space [12]. In a quadrupole channel which has average axial symmetry, the fluctuations in the two transverse directions have the same magnitude and functional form, and are out of phase by p. The beam envelopes can then be written as xm = xm0 + dxm and ym = xm0 - dxm, where xm0 is nearly independent of s0 and dxm has an average value of zero. The electric field can then be split into a steady-state component and a fluctuating component with a linear expansion in dxm. The resulting fluctuating field component is
![]() (3)
(3)
Using Equation (3), setting Efx = Esfx + Ecfx, where Efx, Esfx and Ecfx are respectively the x components of the fluctuating parts of the effective focusing field, the space charge field and the focusing field, and solving for dxm, gives
![]() (4)
(4)
in which e (by definition) is the dielectric constant. The dielectric constant for this case is
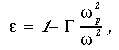 (5)
(5)
in which G = 1/2, wp = (q2ns/e0gm)1/2 is the plasma frequency, and ns is the particle number density. Equation (5) will be used for other types of beams and for halos with different values for G, depending on the geometry.
In deriving Equations (4) and (5) it was assumed that wp2/w2 << 1, and that fluctuations in the focusing fields and space charge fields occur sinusoidally with the same frequency. For most focusing channels the fluctuating component of the focusing field is not a sinusoidal function of longitudinal distance along the channel. In order to define the dielectric constant, the fluctuations are approximated as sinusoidal functions of s0. Small deviations in the functional form are assumed not to have a significant effect on the dielectric response of the beam.
Since e<1, Equation (4) represents an enhancement of the periodic focusing field. This effect results from the fact that the beam has maxima in its extent along any axis, and minima in the magnitude of its space charge field, at longitudinal positions along the channel where the focusing field along that axis is at a maximum. Likewise, the beam has maxima in the magnitude of its space charge field where the focusing field is at a minimum. Fluctuations in the space charge field are therefore correlated with the focusing so that they enhance the effective focusing field.
Substituting Equation (4) into Equation (2) leads to the conclusion that the effect of the dielectric response of the beam is to increase the effective transverse focusing field of a quadrupole channel by the factor 1/e2. For example, a continuous beam in a quadrupole channel with wp/w = 0.2 has a dielectric constant of 0.98. The dielectric response increases the effective focusing field of this channel by about 4%.
The same technique can be used to find the effect of the dielectric response on halo particles surrounding the uniform-density core of a continuous beam. The model of a uniform-density continuous beam core that is mismatched in a continuous (nonperiodic) focusing channel has been used to study the evolution of halo particles [5], in which variations in the space charge field resulting from the oscillating core were found to drive some particles to larger radii. Here, the effect on the effective focusing strength is found from oscillations of the space charge fields for a matched beam in a periodic channel. The same result applies to a mismatched beam in a periodic channel if the frequency of the mismatch oscillations is much less than w.
The beam has average axial symmetry, and variations in xm and ym are out of phase by p. The dielectric response of halo particles arises from the periodic motion of the particles relative to the beam axis, and also from the periodic variations in the shape of the core. The position of a halo particle is written as (x, y) = (x0 + dx, y0 + dy), and the envelopes are again xm = xm0 + dxm and ym = xm0 - dxm. Using the electric field along a transverse direction outside of a continuous, uniform-density elliptic beam core [12], the self electric field can be written in terms of a steady-state component and a fluctuating component with linear expansions in the fluctuating quantities. Solving for the resulting particle motion by the same method as in the previous case, the fluctuating field is again described by Equations (4) and (5). In this case
 (6)
(6)
For example, with x0 = 1.5xm0 and y0 = 0, wp/w = 0.2 gives a dielectric constant of approximately 0.99. The dielectric response increases the effective focusing field at this location by about 2%.
The same method will now be used for the core of a bunched beam with average axial symmetry, which is taken as a uniform-density ellipsoid. The envelope fluctuation in the longitudinal direction is typically either out of phase with the transverse fluctuations by p/2 or it has a different (and nonresonant) frequency from the transverse fluctuations; either way it can be ignored in finding the effective transverse focusing. The electric field in a transverse direction inside a uniform ellipsoid without images [12] can be split into a steady-state component and a fluctuating component with a linear expansion in dxm. The remaining integral is solvable analytically, resulting in a fluctuating field component of
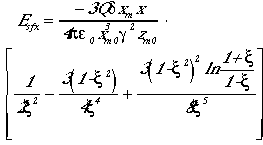 (7)
(7)
where x = (1-xm02/g2zm02)1/2 is the eccentricity of the bunch in the beam frame, zm is the beam envelope in the z direction and Q is the total charge of each bunch. For a bunch that is spherical in the beam frame (gzm0=xm0), Equation (7) becomes Esfx = -3Qdxmx/(10pe0xm04). The same method as in the previous cases results again in Equations (4) and (5), in which G equals the quantity in square brackets in Equation (7). For the special case in which the bunch is spherical in the beam frame, G = 0.4. For example, a beam with an aspect ratio of gzm0/xm0 = 2 (for which G is approximately 0.4) in a quadrupole channel with wp/w = 0.3, has a dielectric constant of approximately 0.964. The dielectric response increases the effective focusing field of this channel by about 8%.
The envelope equations [13] can be used to relate wp/w to the transverse space charge tune depression (kx/kx0) and the phase advance per period (sx0), giving
 (8)
(8)
in which gr = 1-gxm2/2g2zm2 is the radial geometry factor [14]. g is the geometry factor, which is a function only of the aspect ratio of the bunch, gzm/xm, when image fields are negligible; it is a function also of the pipe radius when image fields are significant [14]. Without image fields, g can be approximated as 2gzm/3xm when 1 <= gzm/xm <= 4 with about 10% accuracy. Equation (8) applies for a continuous beam with gr = 1. The first example of a continuous beam with wp/w = 0.2 could therefore correspond to kx/kx0 = 0.5 and sx0 = 590. The example of a bunched beam with gzm/xm = 2 and wp/w = 0.3 could correspond to kx/kx0 = 0.5 and sx0 = 800.
Two uniform-density beams with the same energy, current, space charge tune depression, and aspect ratio will have different matched beam properties if one is in a periodic quadrupole channel and one is in a channel with continuous focusing, if both channels have the same effective focusing strength in the absence of space charge. With space charge, the effective focusing strength of the periodic channel is increased over that of the continuous channel, resulting in a smaller phase advance per period, a higher average beam density and a smaller average beam radius.
Reducing the frequency of the focusing increases the dielectric response and increases the effective focusing strength for a uniform-density beam, but also results in greater oscillations of the matched beam envelope. For applications in which current loss into the conducting channel is an important factor, the increase in the magnitude of the envelope oscillations as the focusing frequency is decreased could lead to greater particle losses even as the effective focusing field on the beam core is enhanced.
This work is supported by G. H. Gillespie Associates, Inc., internal research and development funds.
[1] M. Reiser, Part. Accel. 8, 167 (1978), and references therein.
[2] M. Reiser, J. Appl. Phys. 52, 556 (1981), and references therein.
[3] C. L. Bohn, Phys. Rev. Lett. 70, 932 (1993).
[4] T. P. Wangler, Proceedings of the 1993 Computational Accelerator Physics Conference, edited by R. Ryne (American Institute of Physics, New York, 1993), p9.
[5] R. L. Gluckstern, Phys. Rev. Lett. 73, 1247 (1994).
[6] J. M. Lagniel, Nucl. Inst. Methods Phys. Res., Sect. A 345, 46 (1994).
[7] R. A. Jameson, Advanced Accelerator Concepts, edited by J. Wurtele (American Institute of Physics, New York, 1993), p969.
[8] K. Avinash, A. K. Agarwal, M. R. Jana, A. Sen and P. K. Kaw, Phys. Plasmas 2, 3569 (1995).
[9] N. Brown, "Dielectric Response of Particle Beams to Periodic Focusing," accepted for publication in Physical Review E (August 1996).
[10] G. Schmidt, Physics of High Temperature Plasmas (Academic, New York, 1979), p47.
[11] M. Reiser, Theory and Design of Charged Particle Beams (Wiley, New York, 1994), Section 4.4.
[12] O. D. Kellogg, Foundations of Potential Theory (Dover, New York, 1953), p194.
[13] Reference 11, Section 5.4.11.
[14] C. K. Allen, N. Brown and M. Reiser, Part. Accel.
45, 149 (1994).